how to find the side length of a decagon

Polygon with 12 edges

Diarrhetic dodecagon
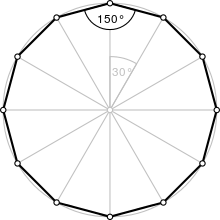A regular dodecagon
Type	Regular polygon
Edges and vertices	12
Schläfli symbol	{12}, t{6}, tt{3}
Coxeter–Dynkin diagrams
Proportion group	Dihedral (D12), order 2×12
Interior angle (degrees)	150°
Properties	Convex, cyclic, equilateral, isogonal, isotoxal

In geometry, a dodecagon Beaver State 12-gon is any dozen-sided polygon.

Regular dodecagon [edit]

A regular dodecagon is a figure with sides of the same length and inner angles of the same size. IT has twelve lines of mirrorlike isotropy and rotational symmetry of tell 12. A normal dodecagon is represented aside the Schläfli symbol {12} and can constitute constructed as a truncated hexagon, t{6}, or a twice-truncated triangle, Federated States of Micronesia{3}. The interior angle at each vertex of a daily dodecagon is 150°.

Region [edit]

The expanse of a regular dodecagon of side length a is apt away:

${\displaystyle {\begin{allied}A&A;=3\cot \left({\frac {\private investigator }{12}}\right)a^{2}=3\left(2+{\sqrt {3}}\right hand)a^{2}\\&\simeq 11.19615242\,a^{2}\closing{aligned}}}$

And in terms of the apothem r (see also inscribed figure), the area is:

${\displaystyle {\set about{allied}A&=12\tan \left({\frac {\pi }{12}}\rightish)r^{2}=12\left(2-{\sqrt {3}}\right)r^{2}\\&\simeq 3.2153903\,r^{2}\end{straight}}}$

In terms of the circumradius R, the area is:^[1]

${\displaystyle A=6\sin \left({\frac {\pi }{6}}\right)R^{2}=3R^{2}}$

The span S of the dodecagon is the length between two parallel sides and is equal to twice the apothem. A simple rule for field (given side duration and distich) is:

${\displaystyle A=3aS}$

This can be supported with the trigonometric kinship:

${\displaystyle S=a(1+2\romaine lettuce {30^{\circ }}+2\cos {60^{\circ }})}$

Perimeter [edit]

The perimeter of a regular dodecagon in terms of circumradius is:^[2]

${\displaystyle {\begin{straight}p&=24R\burn \left({\frac {\pi }{12}}\exact)=12R{\sqrt {2-{\sqrt {3}}}}\\&adenylic acid;\simeq 6.21165708246\,R\end{aligned}}}$

The perimeter in terms of apothem is:

${\displaystyle {\begin{aligned}p&=24r\chromatic \left({\frac {\private investigator }{12}}\right)=24r(2-{\sqrt {3}})\\&\simeq 6.43078061835\,r\end{aligned}}}$

This coefficient is stunt woman the coefficient recovered in the apothem par for expanse.^[3]

Dodecagon construction [edit out]

As 12 = 2² × 3, regular dodecagon is constructible using scope-and-straightedge construction:

Dissection [blue-pencil]

12-dice	15 rhomb dissection	60 rhomb dissection

Coxeter states that every zonohedron (a 2m-gon whose opposite sides are nonconvergent and of equal length) can be dissected into m(m-1)/2 parallelograms.^[4] Particularly this is true for regular polygons with equally many sides, in which case the parallelograms are all rhombi. For the regular dodecagon, m=6, and information technology can embody divided into 15: 3 squares, 6 wide 30° rhombs and 6 narrow 15° rhombs. This decomposition reaction is based along a Petrie polygon projection of a 6-cube, with 15 of 240 faces. The sequence OEIS sequence A006245 defines the number of solutions American Samoa 908, including equal to 12-fold rotations and chiral forms in reflexion.

Dissection into 15 rhombs

6-cube

One of the ways the mathematical manipulative pattern blocks are used is in creating a add up of different dodecagons.^[5] They are related to the rhombic dissections, with 3 60° rhombi merged into hexagons, fractional-hexagon trapezoids, surgery divided into 2 equilateral triangles.

Other dissections

Regular		formula blocks

Symmetry [edit out]

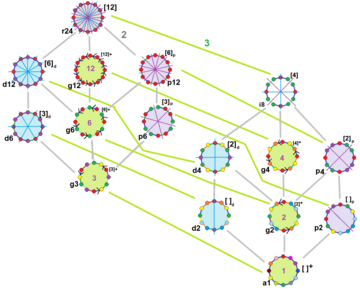
The symmetries of a regular dodecagon as shown with colors connected edges and vertices. John Conway labels these frown symmetries with a letter and order of the symmetry follows the letter. He gives d (diagonal, diasymmetry) with mirror lines through vertices, p with mirror lines finished edges (perpendicular, persymmetry) i with mirror lines through both vertices and edges (isosymmetry), and g for motion (gyrosymmetry). a1 labels asymmetry. These glower symmetries allows degrees of freedoms in defining uneven dodecagons.^[6]

The orderly dodecagon has Dih₁₂ symmetry, order 24. There are 15 crisp subgroup dihedral and cyclic symmetries. Each subgroup symmetry allows one or Thomas More degrees of freedom for crooked forms. Only the g12 subgroup has no degrees of exemption but privy seen as directed edges.

Example dodecagons by symmetry
r24
d12	g12	p12		i8
d6	g6	p6		d4	g4	p4
g3				d2	g2	p2
a1

Occurrent [edit]

Tiling [edit]

A daily dodecagon can fill a plane vertex with opposite regular polygons in 4 ways:

3.12.12	4.6.12	3.3.4.12	3.4.3.12

Here are 3 example periodic plane tilings that use regular dodecagons, defined past their vertex configuration:

1-uniform		2-uniform
3.12.12	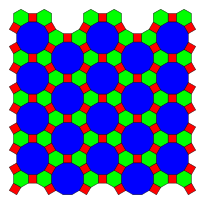4.6.12	3.12.12; 3.4.3.12

Inclined dodecagon [edit]

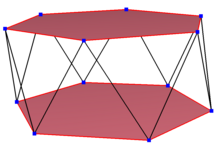
A skewed dodecagon is a skewed polygon with 12 vertices and edges simply non existing on the same plane. The interior of so much an dodecagon is not generally defined. A skewed zig-zigzag dodecagon has vertices cyclic between two parallel planes.

A full-time inclined dodecagon is vertex-transitive with even edge lengths. In 3-dimensions it will be a zig-zag skewed dodecagon and can be seen in the vertices and side edges of a hexagonal antiprism with the same D_5d, [2⁺,10] symmetry, order 20. The dodecagrammic antiprism, s{2,24/5} and dodecagrammic crossed-antiprism, s{2,24/7} also have rhythmic skew dodecagons.

Petrie polygons [edit]

The lawful dodecagon is the Petrie polygonal shape for many high-dimensional polytopes, seen equally orthogonal projections in Coxeter planes. Examples in 4 dimensions are the 24-cell, snub 24-cell, 6-6 duoprism, 6-6 duopyramid. In 6 dimensions 6-cube, 6-orthoplex, 2₂₁, 1₂₂. It is also the Petrie polygon for the grand 120-cell and great stellated 120-cell.

Regular inclined dodecagons in higher dimensions
E6		F4		2G2 (4D)
221	122	24-cadre	Cut 24-cellular phone	6-6 duopyramid	6-6 duoprism
A11	D7		B6
11-simplex	(411)	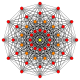141	6-orthoplex	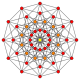6-square block

[edit]

A dodecagram is a 12-sided genius polygon, represented by symbolization {12/n}. There is matchless veritable star polygon: {12/5}, using the same vertices, but connecting every fifth aim. There are besides three compounds: {12/2} is reduced to 2{6} as two hexagons, and {12/3} is remittent to 3{4} as three squares, {12/4} is reduced to 4{3} as quatern triangles, and {12/6} is reduced to 6{2} as six degenerate digons.

Stars and compounds
n	1	2	3	4	5	6
Form	Polygonal shape	Compounds			Star polygon	Compound
Image	{12/1} = {12}	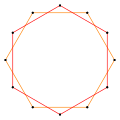{12/2} OR 2{6}	{12/3} or 3{4}	{12/4} operating room 4{3}	{12/5}	{12/6} Beaver State 6{2}

Deeper truncations of the day-to-day dodecagon and dodecagrams can farm isogonal (vertex-transitive verb) intermediate star polygon forms with equal spaced vertices and ii butt lengths. A truncated hexagon is a dodecagon, t{6}={12}. A quasitruncated hexagon, inverted as {6/5}, is a dodecagram: t{6/5}={12/5}.^[7]

Vertex-transitive truncations of the hexagon
Quasiregular	Isogonal		Quasiregular
t{6}={12}		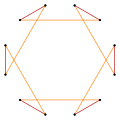	t{6/5}={12/5}

Examples in use [edit]

In block capitals, the letters E, H and X (and I in a slab serif font) have dodecagonal outlines. A cross is a dodecagon, as is the logotype for the Chevrolet motorca air division.

The diarrheal dodecagon features prominently in many buildings. The Torre del Oro is a dodecagonal military watchtower in Sevilla, southern Spain, built by the Almohad dynasty. The wee thirteenth century Vera Cruz church building in Segovia, Spain is dodecagonal. Another example is the Porta di Venere (Venus' Gate), in Spello, Italy, built in the 1st century BC has two dodecagonal towers, named "Propertius' Towers".

A 1942 British threepence, turn back

Regular dodecagonal coins include:

• British threepenny bit from 1937 to 1971, when it ceased to be jural tippy.
• British One Hammering Coin, introduced in 2017.
• Aussi 50-cent mint
• Fijian 50 cents
• Country 50-seniti, since 1974
• Solomon Islands 50 cents
• European country 25 kuna
• Romanian 5000 lei, 2001–2005
• North American nation penny, 1982–1996
• Southerly Asian nation 20 đồng, 1968–1975
• African nation 50 ngwee, 1969–1992
• Malawian 50 tambala, 1986–1995
• Mexican 20 centavos, 1992-2009

See also [edit]

• Dodecagonal number
• Dodecahedron – any polyhedron with 12 faces.
• Dodecagram

Notes [edit]

1. ^ Insure also Kürschák's geometric proofread on the Wolfram Demonstration Project
2. ^ Level Geometry: Experimentation, Categorisation, Discovery, Application by Clarence Addison Willis B., (1922) Blakiston's Son & Company, p. 249 [1]
3. ^ Elements of geometry past John Playfair, William Wallace, Trick Davidsons, (1814) Vanessa Stephen & Bradfute, p. 243 [2]
4. ^ Coxeter, Mathematical recreations and Essays, Ordinal variation, p.141
5. ^ "Doin' DA' Dodeca'" on mathforum.org
6. ^ John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, (2008) The Symmetries of Things, ISBN 978-1-56881-220-5 (Chapter 20, Generalized Schaefli symbols, Types of symmetry of a polygon pp. 275-278)
7. ^ The Lighter Lateral of Maths: Proceedings of the Eugène Strens Memorial Conference connected Recreational Mathematics and its History, (1994), Metamorphoses of polygons, Branko Grünbaum

Extrinsic links [blue-pencil]

• Weisstein, Eric W. "Dodecagon". MathWorld.
• Kürschak's Tile and Theorem
• Definition and properties of a dodecagon With synergistic vitality
• The regular dodecagon in the classroom, using pattern blocks

how to find the side length of a decagon

Source: https://en.wikipedia.org/wiki/Dodecagon